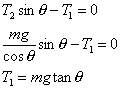Static Equilibrium

The word static means not changing. Sometimes we don’t want an object to move, like when we are designing a bridge or a building. Newton’s second law says that an object will accelerate if there are unbalanced forces on it, therefore the way to prevent acceleration is to make sure that there are no unbalanced forces, or in other words, Fnet
= 0.

Preventing acceleration, however, is not enough. We also want to keep the object from rotating, and this means preventing angular accelerations, which are cause by a non-zero net torque. Thus the conditions for static equilibrium are

1. Sum of external forces = 0

2. Sum of torques about any axis = 0

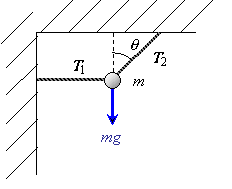
The sum of torques must be zero about any axis, because you don’t want angular acceleration about any axis. Also, if the object is not rotating, then there is no axis of rotation in the first place. This freedom in choosing the axis of rotation is very helpful in solving static equilibrium problems.

Examples

Sum of Forces Only

This example uses only the first condition. There are no torques involved. Suppose a mass m is supported by two ropes, as shown below. One rope extends horizontally from the wall, and the other rope goes to the ceiling at an angle q from the vertical. The problem is to calculate the tension in each rope, so that you can be sure to use a strong enough rope.

We begin by making a free-body diagram of the mass m. This is a picture that isolates the mass from its surroundings, and shows only those forces actin on the mass. The forces acting on this mass are the tensions in the two ropes, which we will call T1 and T2, and its weight mg, which of course points down:

We will also need to break the T2 vector into x and y components (the other two force vectors are already on either the x or y axis). This becomes

Now, for the total force to add up to zero, all the vectors along the y axis and all the vectors along the x axis must add up to zero.

1. Sum of forces in the x-direction equals zero

T2 sin q – T1 = 0

2. Sum of forces in the y-direction equals zero

T2 cos q – mg = 0

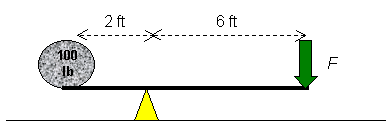
We now have two equations in two unknowns, T1 and T2 (we are assuming that m and q are known). The second equation can be solved for T2 to give

This can be substituted into the first equation, which can then be solved for T1:

Sum of Torques Only

This example uses only the second condition. Suppose you want to lift a 100-lb. rock using an eight-foot pole. You place the fulcrum so that it is two feet away from the rock and six feet from the end where you are pushing. What is the minimum weight you need to
put on your end of the pole to hold up the rock?

If we take the fulcrum as the axis of rotation, then there are two torques. The weight of the rock is trying to rotate the pole counter-clockwise, and your weight on the other end of the pole is trying to rotate it clockwise. When the system is just in balance, these two opposing torques will be equal. Since torque is force times lever arm, we have

(100 lb.)(2 ft) = (F)(6 ft)

which can be solved to give

F = 33 lb.

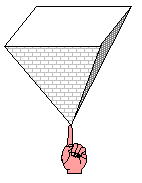
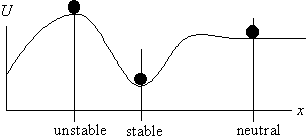
Levers and Pulleys: Mechanical advantage

The previous example is a lever. We will have more to say about levers, pulleys, and other simple machines after we discuss work and energy.

Stability

Static equilibrium is not always stable. For example, an upside-down pyramid exactly balanced on its point is theoretically in static equilibrium, but if it moves even a microscopic distance away from that balance point, it will fall.

The test for stability is to see what happens if the system is moved a small amount (or perturbed) away from the stable position. If forces arise that tend to push it back toward the stable position, then it is a stable equilibrium. If forces arise that tend to push it farther away from the stable position, then it is an unstable equilibrium. If no forces occur either way, then it is neutral.

A simple test is to look at the change in potential energy. A system will tend to minimize its potential energy, so if the perturbation causes an increase in potential energy (as seen by its center of mass rising), then it is a stable  configuration. On the other hand, if a perturbation causes the center of mass to lower, decreasing the potential energy, then the system is unstable.

Stability and the Potential Curve