| Work is one of those words that physics borrows from the everyday language, redefining it to suit its own specialized needs. The physics definition is a precise mathematical statement, which does not always reflect the common usage of the term. For example, we say someone is “working” whenever they are at their job. They may or may not be doing work in the physics sense, depending on what their job is.
The common usage of work is closest to the physics definition when we talk about physical labor. Imagine that you are helping a friend move, and you have just lifted a heavy dresser into the back of a truck. You might say “Wow, that was work!”, and in this case the physicist would agree. After lifting the dresser into the truck, you are tired: the effort cost you energy. Through the force of your muscles you have transferred some of your personal supply of energy to the dresser. In general, work is a transfer of mechanical energy, such as from you to the dresser, and the numerical value of the work done is equal to the amount of energy that was transferred. However, we do not yet have a proper definition of energy, relying instead on your intuitive notion of it. What we are going to do is give a precise mathematical definition to work, and then use work to define what we mean by energy. We hope that this definition of energy will mostly agree with your intuitive idea. We can alter the mechanical situation of an object by changing its position, its velocity, or both. To change its velocity will require a force. To change its position (without changing its velocity) will also require a force if there are any other forces such as gravity or friction acting on the mass. In order for this force to have any effect on the mass it must be exerted over some distance, and so we propose that
We expect that the amount of work increases if either the force or the distance increases, so the simplest formula would be Work = Force ´ distance:
This implies that the units of work are newtons times meters. This unit has its own name and is called a Joule (J):
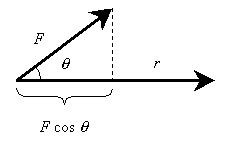
A joule is actually not much energy at all. It is the energy that it would take to exert a force of one newton over a distance of one meter, and a newton is a rather small force (recall that a kilogram weighs 9.8 N, so a newton is about the weight of a tenth of a kilogram, or about 100 grams). If you imagine lifting a kilogram from the ground to a tabletop (about one meter), then you have done about ten joules of work. This definition of work, however, is not quite complete. In fact it makes no sense at all unless the force is constant over the interval, but motion with a varying force requires calculus, and so we will limit our discussion to constant forces. What if the applied force was perpendicular to the displacement? Then that force obviously had nothing to do with that displacement. It’s like if several people are helping to push a car but for some reason one confused person is pushing sideways on the driver door. That person’s effort would not help (or hinder) the movement of the car, and that person could not claim to have contributed to the work done on the car. So, we that the force in our formula refer only to forces in the direction of motion. If the force is at an angle we must resolve it into vector components parallel and perpendicular to the motion, and use only the component along the direction of motion to calculate work. So now we have
In vector notation the displacement is represented by the vector r, and if q is the angle between F and r then the component of force in the direction of r will be F cos q, so
Note that W can be negative. It is negative whenever the force is directed opposite to the motion. This will happen if you are trying to slow down a moving body. To slow it down you must exert a force opposite to the direction of motion, and you are then doing negative work on it, which means that you are taking energy away from it instead of giving it more energy. We originally stated that work was a transfer of energy. When you do positive work on a body, you are increasing its energy, and when you do negative work on it you are reducing its energy. When you exert a force to slow down a moving object you taking energy away from it, and when you speed it up you are giving it more energy.
At the risk of inviting more confusion, we can also talk about just the amount of work that we have done against just one particular opposing force, even when several forces are present. Suppose that you are pushing a box up a ramp. You are doing work to overcome the opposing forces of both gravity and friction, and you might want to know how much of yourwork is being used just to overcome gravity. All you have to do is to figure out how much of your force is going toward overcoming the particular force you are interested in, such as gravity, and then you can figure the corresponding work from force times distance. Forexample, if the force of gravity has a component of –mg sin q along the ramp, then you must counter it with an equal and opposite force of mg sin q, and so the work you do against gravity after pushing it a distance d is just mgd sin q. Question: How much work is done against gravity when you push a box across the floor? The answer is none. You are doing work against the force of friction, which directly opposes the motion, but you do no work against gravity because your pushing force is perpendicular to the gravitational force. Question: How much work do you do against gravity when you lift an object up and put it back down again? Once more the answer is zero, but for a different reason. When you lift the object up, both your force and the displacement are in the up direction and so the work you do is positive. When you put it back down, you are still exerting an upward force to keep it from falling, but the displacement is downward and hence the work you do on the object is negative. As we shall see shortly when we study gravitational potential energy, the positive and negative work that you do are equal in magnitude and so the total work done by you against gravity is zero for a round trip. So all those bench presses you’ve done in your life didn’t involve any work? Of course they did, but the work was done against the internal resistance of your own muscles, and not against gravity. If your muscles could somehow capture the energy extracted while the weight was being lowered and re-use it to raise the weight again, you could lift weights all day long and never get tired (imagine that you had springs for arms). Unfortunately, we can’t do this. Your muscles discard the surplus energy in the form of heat, and they have to use “new” energy each time you lift the weight. Hence you get tired (and hot). Notice that in talking about the work that you do, we looked at the direction of the force that you exert on the object and not the direction of the force that gravity exerts on it. We are not presently interested in the work that gravity did on the object. We are looking for the work that you did on the object in order to overcome the force of gravity, and so it is your force that counts. Work: Key Points
|