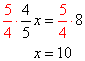Multiplication Principle
Multiplying (or dividing) the same non-zero number to both sides of an equation does not change its solution set.
Example:
![]()
so if 6x = 12, then 18x = 36 for the same value of x (which in this case is x = 2).
The way we use the multiplication principle to solve equations is that it allows us to isolate the variable by
getting rid of a factor that is multiplying the variable.
Example: 2x = 6
To get rid of the 2 that is multiplying the x, we can divide both sides of the equation by 2, or multiply by its reciprocal (one-half). Either divide both sides by 2:
![]()
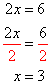
or multiply both sides by a half:
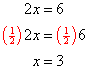
- Whether you prefer to think of it as dividing by the number or multiplying by its reciprocal is not important, although when the coefficient is a fraction it is easier to multiply by the reciprocal:
Example:
![]()
Multiply both sides by the reciprocal of the coefficient, or ![]()